Fundamental application
Looking at a colony of bacteria under the microscope expressing fluorescent proteins in an oscillating manner sounds like fun, but the oscillating system might have real applications. One hypothetical application that could be used in fundamental research involves interactions between genes. To become familiar with this hypothetical application, an example is given:
Say, there exists a particular gene A, which might interact with other genes B, C & D, but there is no evidence for this. To get an idea about this interactive behaviour, gene A can be knocked out. Now that gene A is knocked out it is desirable to find out what happened with genes B, C & D. One way is looking at the transcriptional level of those genes, to see whether there are differences compared to the normal situation. Another is measuring the concentrations of the proteins that are expressed by gene B, C & D. By looking at those data, conclusions can be drawn. For example, gene B seems to be upregulated, gene C seems to be downregulated and nothing happened to gene D. Now it seems that gene A interacts with both genes B & C, but there is still no evidence for that, as there is a dozen of other factors that could have influenced the expression of genes B & C. Think of growth conditions, growth phase, other gene interactions, etc.
Using the oscillating system, it is possible to have gene A oscillating and at the same time look at what happens to the other genes B, C & D through time. If it appears that gene B shows changes in its expression level pattern or protein concentration pattern and genes C & D do not show a difference compared to the normal situation whilst gene A is oscillating, it means that there is evidence for the fact that gene A is interacting with gene B and not with gene C & D.
As there are some genes that cannot be knocked out, because without it the organism cannot survive, the use of knockouts is limited. The oscillating system can get the expression level of a crucial gene E very low at certain time points while it makes up for the loss at other time points where it is over-expressed. This means that, by looking at changing expression patterns of other genes over time, it is possible to get interactional information for gene E that until now was very hard to study.
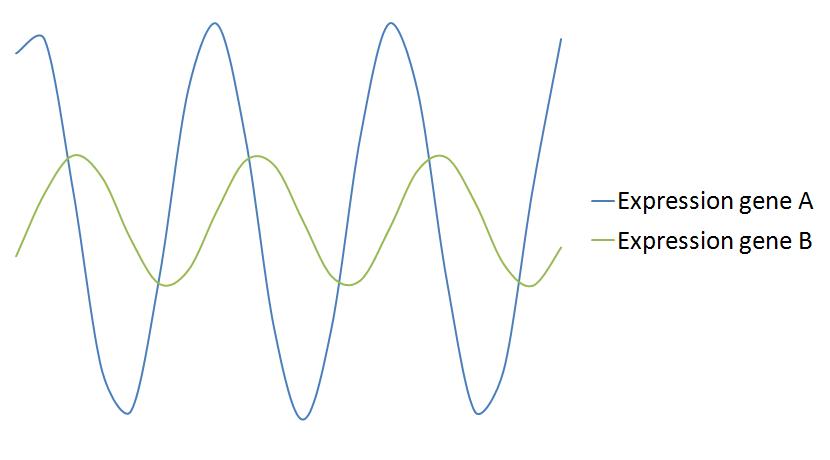
Next to that, using the oscillating system, it would be possible to look at rates of interaction between genes. Imagine that gene B is regulated by gene A, which is oscillating. If the interaction speed between A & B would be extremely fast, it might be possible that gene B starts oscillating together with gene A. There should be a delay however. By looking at this delay and the resulting pattern of gene B, conclusions can be drawn about the rate of interaction between both genes. The following figure illustrates what it could look like:
Fig. 1 A hypothetical graph of the expression levels of genes A & B over time
Industrial application
The oscillatory system could also be used in industrial applications. One hypothetical application involves ranging enzyme concentrations. If for example a particular protein is manufactured by carrying out two different reactions in separate tanks by using an organism with an over-expressed enzyme A in tank 1 and another strain with over-expressed enzyme B in tank 2, the same follow-up of reactions can be carried out in one tank using two oscillatory systems present in a single organism. For example, a particular subset of enzymes can be produced in an oscillating manner so that the product is exposed to a high concentration of enzyme A at a certain time point and a high concentration of enzyme B at another time point. This way the efficiency of such a process could be increased.
 "
"